F-Theta Lenses Tutorial


Please Wait
F-Theta Lenses
F-theta lenses have been engineered to provide the highest performance in laser scanning or engraving systems. These lenses are ideal for engraving and labeling systems, image transfer, and material processing. For many applications in laser scanning and engraving, a planar imaging field is desired for the best results. A spherical lens can only image along a circular plane (see Fig. 1 A). The flat-field scanning lens solves this problem. However, the displacement of the beam depends on the product of the effective focal length (f) and the tangent of the deflection angle θ [f × tan (θ), see Fig. 1 B].
While this nonlinear displacement can be accounted for with the proper software algorithm, the ideal solution is to produce a linear displacement (i.e., constant scan rate). F-theta lenses are designed with a barrel distortion that yields a displacement that is linear with θ (f*θ, see Fig. 1 C). This simple response removes the need for complicated electronic correction and allows for a fast, relatively inexpensive, and compact scanning system.

Click to Enlarge
Figure 1 - Scanning Lenses
F-theta lenses solve many of the problems associated with laser scanning. Additionally, the compact design of the f-theta lens allows the user to reduce the number of optical components needed to provide a flat image plane. These lenses are able to realize tighter spot sizes, which translate into higher resolution for scanning or printing, as well as higher intensity for engraving or welding. Most importantly, the spot size (resolution and intensity) are nearly constant across the entire image plane.
Scan Lens Setup
Laser scanning systems are optimized for precision control of a laser’s beam waist size (diameter of the focused spot) and the accurate position of that waist. Typically a laser scanning system will incorporate one or two scan mirrors, depending on function. For instance, in a single mirror system, the mirror will be placed at the entrance pupil position of the f-theta lens. In a two mirror system, the entrance pupil of the f-theta lens is placed between the two mirrors. To maximize the performance of the f-theta lens, the mirror separation should be minimized.
Scan Lens Characteristics
Some of the most important factors to consider when selecting a f-theta lens are operating wavelength, spot size, and scan field diameter (SFD). Through these parameters, the user may then place further constraints upon a scanning system such as entrance beam diameter, scanning mirror deflection, mirror placement, and mirror position.
Scan Field Diameter (SFD), or Scan Length, is the diagonal length of a square area in the image plane where the beam can be focused by the lens. This specification helps define deflection (along with the focal length). Output Scan Angle (OSA) is the angle between the output laser beam after it has passed through the scan lens and the normal of the image plane. The OSA will vary across the image field, although for scanning or engraving the change in OSA will not largely impact dynamics. It should be noted that the OSA is always zero for telecentric lenses. Back Focal Length (BFL) is the distance between the apex of the physical lens (outer glass element) to paraxial focus point. Back Working Distance (BWD) is simply the distance between the housing of the lens to the paraxial focus point.
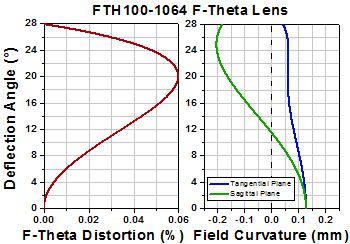
Another important parameter to consider is field distortion and curvature. While f-theta lenses are well designed to provide a flat image plane, a real lens rarely measures up to the theoretical. There will be some amount of distortion and curvature. Figure 2 shows these parameters for our FTH100-1064 f-theta lens, where focal length is 100 mm and the maximum deflection angle is 28°. The figure shows both the field curvature in millimeters and f-theta distortion in percent as a function of scan angle. Typically in constructing a scanning system, it is best to place the zero-curvature point at the midrange of the scan to help limit the amount of curvature realized during the scan.
Summary
As stated previously, the goal of the laser system is to produce a spot size appropriate for the necessary resolution and to accurately position that spot anywhere in a flat image plane. Diffraction-limited scanning lenses, in general, will produce a spot size given by
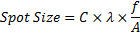
where Spot Size is the 1/e2 beam diameter, λ is the wavelength of the laser, f is the effective focal length of the lens, and A is the entrance beam diameter. C is a constant that relates to the degree of pupil illumination and input truncation (for a Gaussian beam, C = 1.83 when the entrance beam is truncated at the 1/e2 diameter).
The focal length also influences the diameter of the scan field, which is given by
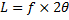
where L is the diagonal of the square scan field, θ is the maximum deflection angle in radians, and again f is the effective focal length of the lens. By maximizing θ, the focal length can be minimized in a system. In general this is the preferred method of maintaining L since it will reduce the necessary size of the optics resulting in a more compact and cost effective system. Additionally, f-theta distortions caused by motor instabilities of the scanning mirrors will be reduced since these distortions scale with the EFL (smaller EFL results in smaller distortions).
| Posted Comments: | |
| No Comments Posted |
 Products Home
Products Home
 F-Theta Scan Lenses
F-Theta Scan Lenses